To play, click "Play" button on the game board above.

Sym-a-Pix rules

Sym-a-Pix is another in a line of Picture Logic puzzles that form whimsical pixel-composed pictures when solved. It is a rotational symmetry puzzle based on a grid with a pixilated picture hidden inside. Using logic alone, the solver determines which squares are painted and which should remain empty until the hidden picture is completely exposed.

Sym-a-Pix puzzles come in B&W and color, and are available in many sizes and difficulty levels taking anywhere from five minutes to several hours to solve. Basic Logic Sym-a-Pix require easy logic techniques and are straightforward to solve while Advanced Logic Sym-a-Pix contain more advanced situations making these puzzles very challenging and rewarding to solve.

Basic Logic Sym-a-Pix

Each puzzle consists of a grid containing dots in various places. The object is to reveal a hidden picture by drawing a block around each dot so that its shape is ‎rotationally symmetrical to the dot, its color matches the color of the dot, there are no other dots ‎inside it and no squares are left out at the end of the solution.‎

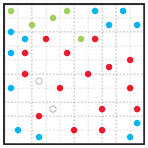
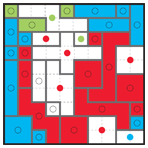
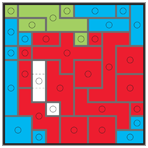
Advance Logic Sym-a-Pix

Each puzzle consists of a grid containing dots in various places. The object is to reveal a hidden picture by drawing a block around each dot so that its shape is ‎rotationally symmetrical to the dot, its color matches the color of the dot, there are no other dots ‎inside it and no squares are left out at the end of the solution.

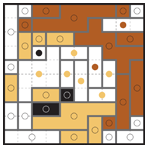
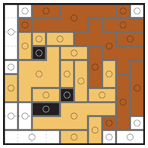
Mega Sym-a-Pix

Each puzzle consists of a grid containing dots in various places. The object is to reveal a hidden picture by drawing a block around each dot so that its shape is ‎rotationally symmetrical to the dot, its color matches the color of the dot, there are no other dots ‎inside it and no squares are left out at the end of the solution.

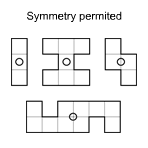
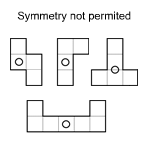
Rotational Symmetry

Rotationally symmetrical block is a shape that looks exactly the same when turning it half circle around the dot forming its axis.

Here are some samples of blocks with and without rotational symmetry: